Getting your Trinity Audio player ready...
Artificial intelligence is rapidly transforming the world, impacting image processing, speech recognition, disease diagnosis, drug development, autonomous transportation, writing, translation, and even the creative production of texts, images, and videos. Now, AI is making its mark in the field of mathematics.
In a new study published in the journal PNAS, Professor Ido Kaminer from the Viterbi Faculty of Electrical and Computer Engineering and student Rotem Elimelech introduced "Ramanujan Machine 2.0," focusing on the relationships between various mathematical constants.
2 View gallery
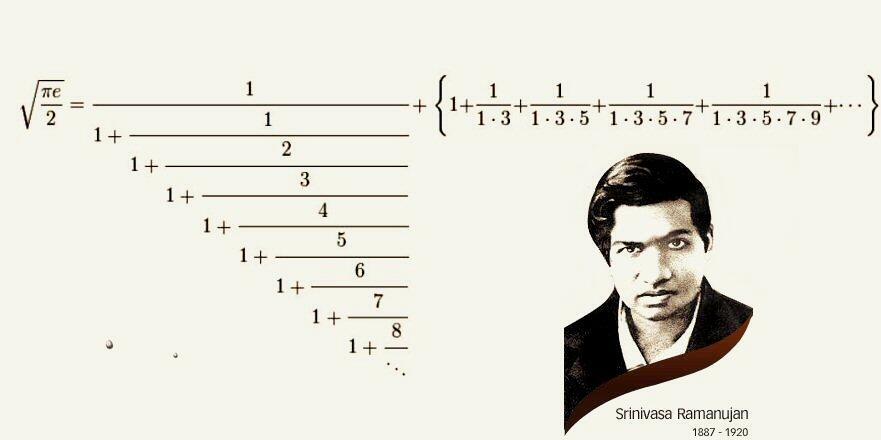

The hypothesis generator is nicknamed the "Ramanjuan machine" after the Indian-born mathematician
(Photo: Technion – Israel Institute of Technology)
This groundbreaking research falls under the domain of AI for Science—advancing scientific knowledge through artificial intelligence. Elimelech and Professor Kaminer, who published a paper about a hypothesis generator they called the "Ramanujan Machine" three years ago, said, "On a philosophical level, our work explores the interaction between algorithms and mathematicians. The new paper demonstrates that algorithms can indeed provide the necessary information to generate creative insights and lead to the discovery of new formulas and relationships between mathematical constants."
The researchers explained that mathematical constants such as pi, the Gauss constant, and the golden ratio are numbers that naturally appear in mathematical developments and have immutable values. These constants hold immense significance not only in mathematics but also in fields like biology, physics, and ecology.
According to the researchers, the work on the original task of discovering new constants yielded several dramatic "by-products":
Demonstrating the effectiveness of "virtual laboratories," where computer simulations mimic "physical" experiments in natural sciences. In these labs, new mathematical formulas and even theorems can be generated using algorithms. Scientific hypotheses drive mathematical research, and the more supporting examples there are, the stronger and more likely the hypothesis is to be correct.
Expanding the use of distributed computing, which relies on numerous computing units (many computers, many processors). The researchers noted that some mathematical discoveries are impossible without the power of distributed computing. In this aspect, they collaborated with Professor Mark Silberstein and utilized a distributed computing infrastructure supported by thousands of volunteers.
The new Ramanujan Machine can assist other researchers by generating search algorithms that serve their purposes. Whenever the algorithm finds a "lead," it can help direct human researchers to examine new phenomena in promising areas.
The study also involved Wolfram Brandt, Carlos de la Cruz Mangual, Ofir David, Professor Mark Silberstein, Yaron Hadad, Rotem Kalish, and Michael Shalit.


